Improve students’ math achievements by understanding their math misconceptions

Identifying students’ math misconceptions is crucial for teachers to help understand why students struggle with specific concepts. By doing so, teachers can identify students in need of extra assistance, and personalize their instruction to address these misconceptions, leading to a deeper understanding of mathematics. This approach also enables teachers to develop more effective teaching strategies and improve students’ overall math achievement.

Math assessment programs can be a valuable tool for teachers to identify students’ common math misconceptions. At NTN, we developed MathKEYmatics, a powerful math assessment program built with features that teachers said they were looking for. One of those features is explicitly labeling misconceptions on intentional distractors that will provide insight on how to cater further instruction to improve student understanding of concepts. Read on to learn more about how understanding misconceptions can be a game-changer in the math classroom.

Common Math Misconceptions

There are a number of common math misconceptions that students may have. Some of these misconceptions include:

  • Misconception: The order of numbers in a division problem does not matter because the order of the numbers can be reversed in a multiplication problem and still get the same product.
    • Reasoning: Students may think that the order of numbers in a multiplication or division problem matters because they are used to seeing numbers written in a certain order. For example, they may know that both 3 x 4 = 12 and 4 x 3 = 12, so will try to apply the same principle to division. In this case, they may think that either 12 4 or 4 12 equals 3 which is incorrect logic.
  • Misconception: When you multiply, the answer is always bigger.
    • Reasoning: Students may transfer what they’ve learned from multiplying whole numbers to multiplying rational numbers. For example, since we know that 3 x 4 = 12, the assumption would be that 3 x 0.4 equals a number greater than 3 when the product is actually equal to 1.2.
  • Misconception: You always have to subtract a smaller number from a larger number.
    • Reasoning: Students may think that you can’t subtract a larger number from a smaller number because they are used to seeing subtraction problems where the larger number is on top and the smaller number is on the bottom. This misconception can result in students experiencing difficulties with regrouping. For example in the problem 304 – 17, a common error would be students subtracting the 4 from the 7 in the ones place rather than regrouping.

How MathKEYmatics Identifies Common Misconceptions

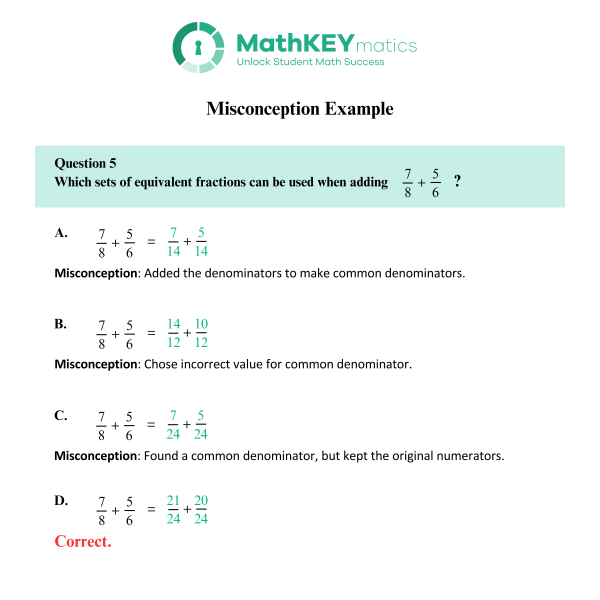Once students complete a math assessment using MathKEYmatics, teachers are provided with real-time data on students’ performance on a variety of math problems. If a student has answered the question wrong because of a common math misconception, it informs the teacher of potential student misunderstanding based on the distractor selected.

Once teachers have identified students’ common math misconceptions, they can better tailor their instruction to address these misconceptions. This may involve providing students with additional instruction on the concept, using different teaching methods, or providing students with opportunities to practice the concept differently.

By gaining insight into student misconceptions, teachers can address the specific misunderstanding rather than completely reteaching the standard.

The team at NTN created MathKEYmatics to ensure math educators were provided with insight into these standard-aligned misconceptions. It is value-priced, so you don’t need to jump through hoops to get it approved, and a turnkey solution to have you up and running in no time.

We are passionate about our partners, and always just a call away. Schedule some time with us to discuss how MathKEYmatics can support math teachers at your school.

Contact Sarah Wood for more information on MathKEYMatics and how it can help your school: https://meet-with-sarah-wood.appointlet.com/s/ntn-mathkeymatics-exploration